There are three types of versines used for the track realignment and rectification methods (Radu, 2003):

• Measured (existing) versine – the versine measured with a real chord on the outer rail of the real existing track. This is the result of the versine (Hallade) survey and based on it are computed the track offsets (slues or slews) required to correct the track geometry.
• Theoretical versineª – the versine not measured but computed using the formula v_i = C²/8R_i , where R_i is the centreline radius at point i. If this point is on a circular curve R is the radius of the curve. If it is on a transition curve R_i = RL/d , where d is the distance from the point i to the beginning of the transition curve and L is the total transition length.

The theoretical versine on a transition curve is:

The versine change dv between consecutive points along the linear transition curve is:

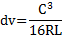
As the name suggest, this type of versine is theoretical, computed presuming a certain chord length but without making any chord offset measurement on the ideal, nondeformed track.

• Ideal versineª – the versine measured as offset to a chord on the ideal track, without any irregularities.

This versine is the same as the theoretical versine if both ends of the chord are on the same geometrical element (straight, circular curve or transition curve).

In the vicinity of the points where the curvature variation changes, the ideal versine is different from the theoretical versine. In the figure below, when measuring the versines for points 2 and 3, the chord is placed on two different alignment elements.

To measure the versine for point 2 the chord is placed between point 1 – on a straight – and point 3 – on the curve. Although not clearly visible in the figure, there is a versine V2 despite the fact that point 2 is actually on a straight, hence the theoretical versine is null.

Similarly for point 3, which requires the chord to be based on point 2 – on the straight – and on point 4 – on the curve? The versine V3, measured to the chord 2-4 is not equal to the circular arc theoretical versine.

Below is another example for a compound curve.

The versine v3 is an ideal one because is measured using the chord 2-4. But if we extend back the curve of radius R2 and find the point 2’ to define the chord length 2’-4, the versine v’3 measured using this chord is equal to the theoretical versine for the curve of radius R2.

When doing a realignment or rectification design using Hallade or other methods, the resulted versines are checked against the ideal versines and not the theoretical ones. If the track lining is checked on site, the measured versine should always be checked against the ideal versine. If the versine of a point on a straight is checked by placing the chord on the adjacent curve it is normal to get, even for an ideal track, a non-null versine.

In the Hallade realignment method (Ellis, 1998; Noblet, 2003) the ideal versines at the start or end of a transition are computed by the following rules:

• When a transition starts or finishes at a half-chord point, the first/last versines are modified by adding/subtracting 1/6 times the full transition rise, dv.
• When the transition starts and finishes exactly midway between two half-chord points the adjustment is equal to 1/48 of the full transition rise, dv. Unless the transition rise is very large, is generally accepted that in this case the rounding off, in Hallade realignment terms, is null.

The justification for these rules will be presented in a future post together with the equations to be considered when the transition curve has an arbitrary position relative to the half-chord points.

Noteª: In some railway literature the labelling of the “theoretical” and “ideal” versines is the other way around – the versines measured on the ideal track are called “theoretical versines”. In fact that’s the way I’ve learned them also. But I chose to use the above way of defining them because it seems now more logical to me. 

The reader familiarised with the other way of defining them is politely asked to excuse this labelling change which does not affect the essence of the subject:  three distinct types of versines – one which is only the result of a mathematical formula and the other two measured on track – either ideal and without irregularities or real, with irregularities and implying also measuring tolerances and errors.

References:

• Cope, G. (1993) British Railway Track – Design, Construction and Maintenance. Permanent Way Institution, Echo Press, Loughborough.
• Noblet, Y. (2003). Épures Hallades. Modification de trace par la méthode des flèches. Available online: http://bazar.perso.free.fr/Files/Other/DOCUMENTATION/Trace/Fleches.pdf
• Ellis, I. (1998). The Hallade Training Manual. Available online: http://www.iainellis.com/hallade.pdf
• Radu, C. (2003) Rectificarea si retrasarea curbelor de cale ferata (Rectification and realignment of the railway track curves). Course notes. Technical University of Civil Engineering Bucharest.